Geometry chapter 1 points lines and planes answers – Geometry Chapter 1: Points, Lines, and Planes Answers unlocks the foundational concepts of geometry, laying the groundwork for a deeper understanding of this fascinating field. Embark on a journey through the intricate world of points, lines, and planes, where clarity and precision converge.

This comprehensive guide delves into the fundamental building blocks of geometry, exploring their properties, relationships, and applications. Prepare to unravel the mysteries of intersections, parallelism, angles, polygons, and more, as we navigate the captivating landscape of geometric principles.

Concepts of Points, Lines, and Planes: Geometry Chapter 1 Points Lines And Planes Answers

In geometry, points, lines, and planes are fundamental building blocks. A point is a location in space with no dimensions. A line is a one-dimensional object that extends infinitely in both directions. A plane is a two-dimensional surface that extends infinitely in all directions.

Points are represented by single letters, such as A, B, and C. Lines are represented by two points, such as AB or CD. Planes are represented by three points, such as ABC or DEF.

Points, lines, and planes have specific properties. Points have no length, width, or height. Lines have length but no width or height. Planes have length and width but no height.

Points, lines, and planes can be found in the real world. Points can represent the location of objects, such as the center of a circle or the vertex of a triangle. Lines can represent the edges of objects, such as the sides of a square or the spokes of a wheel.

Planes can represent the surfaces of objects, such as the top of a table or the floor of a room.

Intersections and Parallelism, Geometry chapter 1 points lines and planes answers

When two lines or planes intersect, they form a point. The point of intersection is the only point that is common to both lines or planes.

Two lines or planes are parallel if they never intersect. Parallel lines or planes lie in the same plane and never cross.

There are several ways to determine if two lines or planes intersect or are parallel. One way is to use the slope of the lines or planes. If the slopes are equal, the lines or planes are parallel. If the slopes are not equal, the lines or planes intersect.

Another way to determine if two lines or planes intersect or are parallel is to use the distance between the lines or planes. If the distance is always the same, the lines or planes are parallel. If the distance is not always the same, the lines or planes intersect.

Angles and Angle Relationships

An angle is formed when two lines or planes intersect. The size of an angle is measured in degrees. A degree is a unit of measurement that is equal to 1/360 of a full circle.

There are different types of angles. Some of the most common types of angles include acute angles, right angles, obtuse angles, and straight angles.

Acute angles are angles that are less than 90 degrees. Right angles are angles that are equal to 90 degrees. Obtuse angles are angles that are greater than 90 degrees but less than 180 degrees. Straight angles are angles that are equal to 180 degrees.

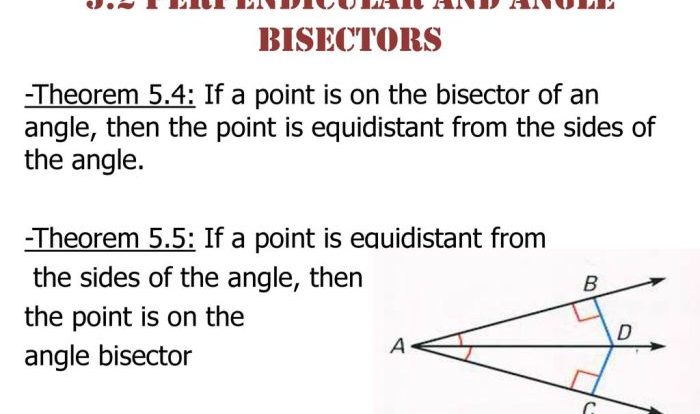
Angles have certain relationships with each other. Some of the most common angle relationships include complementary angles, supplementary angles, and vertical angles.

Complementary angles are two angles that add up to 90 degrees. Supplementary angles are two angles that add up to 180 degrees. Vertical angles are two angles that are opposite each other and share a common vertex.

Question Bank

What are the basic concepts of geometry?

Geometry encompasses the study of points, lines, planes, angles, polygons, and their relationships. It explores the properties, measurements, and interactions of these geometric elements.

How do I determine if lines or planes are parallel?

Parallel lines or planes never intersect, regardless of how far they are extended. To determine parallelism, you can use various methods, such as slope comparison, angle measurement, or the intercept method.

What is the difference between complementary and supplementary angles?

Complementary angles add up to 90 degrees, while supplementary angles add up to 180 degrees. Understanding angle relationships is crucial for solving geometric problems involving angles.